|
CYCLOID : Evolute
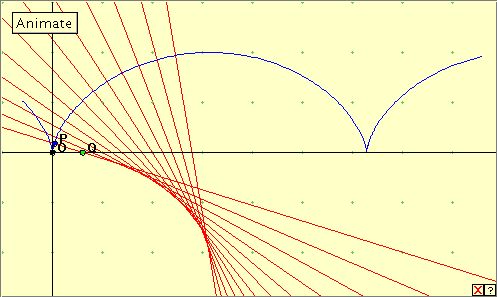
We recall the cycloid as the path traced out by a point on a circle rolling along a straight line. To find the evolute of the cycloid, we draw in the normals to the curve and generate an envelope. Click the linked figure below, and then click the ‘Animate’ button to generate the evolute of the cycloid. Click the button again to stop the generation. You can also see the construction by manually dragging the green point Q along the x-axis. Do you understand the construction? Do you recognize the evolute? Can you name it? describe it?
Bibliography |