Hints and Solutions
To solve these problems, invert the figure with respect to O.
1. Points P, Q, R lie in order on a straight line, and  POR = 90°. Show that the circles PQO, QRO are orthogonal.
POR = 90°. Show that the circles PQO, QRO are orthogonal.
• Line PQR maps to a circle through O with PR as diameter. The two given circles map to perpendicular segments PO, QR (angle in semicircle). Hence the circles are orthogonal.
2. Find the inverse of a set of coaxial circles having O as one of the limiting points.
• We get concentric circles with centre O. For the orthogonal system maps to straight lines through O.
3. Points O, A, B, X lie on a circle. A line through O cuts XA, XB in U, V respectively.
Show that the circles OAU, OVB have a common tangent at O.
• Draw the inverse figure, and show that image segments AU, VB are parallel. (Look at the angles subtended by AO, BO.)
4. BOC is a given angle. A circle through O and B has its centre on OC, and a circle through O and C has its centre on OB. The two circles meet again in X. Show that OX is a diameter of circle OBC.
• Invert to get a kite OCXB with right angles at B and C. Now show BC pperpendicular to OX.
5. (a) By thinking about the properties of inverse points, describe where the image of a circle through O lies under inversion with respect to O.
(b) Points A, B, C lie on a straight line, and O is a point not on that line. Circles OBC, OCA, OAB have centres U, V, W. Prove that the points O, U, V, W lie on a circle. [Hint: You may find it helpful to draw an accurate diagram. Also, remember the Simson line!]
• (a) Let the circle of inversion have centre O, radius 1. If OP is a diameter of the circle to be inverted, and P maps to P' then OP.OP' = 1. If C is the centre of this circle, C maps to C' with OC.OC' = 1. OC = OP/2 implies OC' = 2OP'.

 Simple Cases
Simple Cases 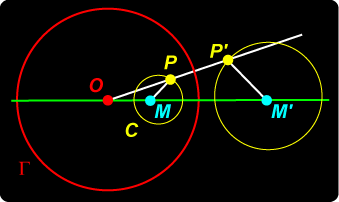 Circle to Circle
Circle to Circle Circle to Line
Circle to Line
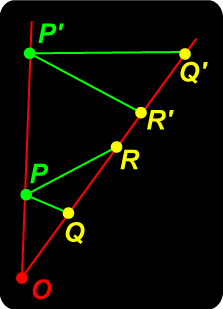 Angle
Angle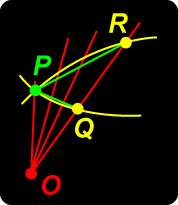 The angle between two smooth curves at a point of intersection is usually defined as the angle between the tangents to the curves at that point. Since the tangent itself is the limit of a set of chords, it will be sufficient for us to show that
The angle between two smooth curves at a point of intersection is usually defined as the angle between the tangents to the curves at that point. Since the tangent itself is the limit of a set of chords, it will be sufficient for us to show that