2. THE NINE-POINT CIRCLE
We have already discovered one circle associated with the triangle – the circumcircle, passing through the three vertices. It is easily seen that it requires just three non-collinear points to determine a circle.
In our triangle ABC we have now discovered two new triplets of points: the midpoints of the sides A', B.', C'; the feet of the altitudes D, E, F. Perhaps there are interesting circles circumscribing these triplets? Let’s try two special cases. You might like to carefully draw your own diagrams. 1. The equilateral triangle Let’s consider the circle through the edge midpoints A', B' , C'. Because this is a very special triangle, a lot of our points coincide! In fact, the circle here becomes the incircle. We look at this later. However, this circle passes through three new easily defined points. Can you describe them? 2. An isosceles triangle See the new diagram. This diagram is more interesting! You might find eight distinct points here which could lie on the circle. What are they? Do the three points found above belong here? Do they lie on the medians or the altitudes? What do you think the nine points might be for a general scalene triangle? |
| You might try a simple experiment. Draw one point in the plane. How many circles can you draw through it? Draw two points in the plane. Can you draw more than one circle through both these points? Would a third non-collinear point determine such a circle? Another idea is to use an algebraic approach. The general equation for a circle is: x2 + y2 + 2fy + 2gx + c = 0 This has three variable coefficients. Inserting the coordinates of three general points (x, y) gives three equations which completely determine these coefficients. |
For a general triangle ABC, as before let A', B', C' denote the midpoints of the sides, D, E, F the feet of the altitudes, and U, V, W the midpoints of the segments joining the orthocentre H to the vertices A, B, C. Then:
Let's use a simpler diagram. Let A'U meet OH in N. Since OA' // AD and UH = OA, we deduce that Finally, since N and U are midpoints of the sides HO, HA of |
Two triangles are called similar if they have the same shape. This occurs if the angles of one are the same as the angles of the other. The symbol ‘ ~’ is used to denote similarity. Two triangles can be shown to be similar by showing that two angles of the first triangle are equal (in size) to two angles of the second triangle. It will then follow that the third pair of angles is equal, since the angles of any triangle sum to 180°. |
Two triangles are said to be congruent if they have the same shape and the same size. In this case, corresponding angles will be equal, and corresponding edges will have the same length. The symbol ‘ There are various tests to determine whether two triangles are congruent.
|
| There is a well-known theorem that in any circle ‘the angle subtended by a chord at the centre is twice the angle subtended at the circumference’.
The proof is simple, with isosceles triangles and external angles. In the case where AB is a diameter of the circle, the angle subtended at the circumference becomes a right-angle. |
1. We know that G trisects OH. Can you show that G also trisects ON? 2. What would be the nine-point circle of 3. Show that AOA'U is a parallelogram, and hence find the position of the midpoint of OU. 4. If 5. Can you find a condition on 6. Do the results of this section still hold when
|
 Exploring
Exploring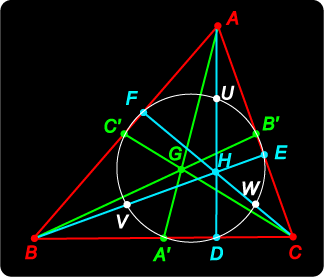 The Nine-Point Circle
The Nine-Point Circle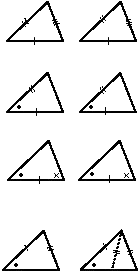 • S.S.S. (Side-Side-Side)
• S.S.S. (Side-Side-Side)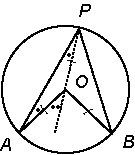 (Wherever did that word ‘subtend’ come from?!)
(Wherever did that word ‘subtend’ come from?!)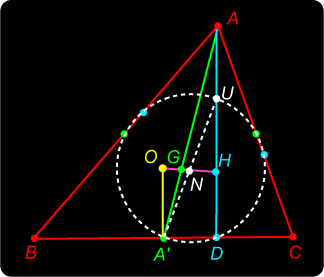 Extensions
Extensions