Fribourg and Hauterive

The Swiss town of Fribourg is a delightful place to visit. It is situated on a rocky spur nearly encircled by a bend of the Sarine River. The river still separates French and German areas of Switzerland. The Lower Town, bristling with church towers still looks very much like a medieval city.
 

About 7 km southwest of Fribourg stands the Cistercian Abbey of Hauterive (‘Oh-ter-eef’), founded in 1138. The Gothic cloisters, added in the years 1320 – 1328, contain a fascinating display of geometric tracery windows. Three sides of the cloisters are still in their original condition, but the south part was removed in the C18th, and two of its windows have been placed on the west and east sides. Each bay of the cloisters contains three Romanesque arches separated by two double columns on the lower level, and then above, either a round or pointed tracery window. It is the round windows which have the most mathematical interest, as they contain a great deal of geometry. It seems clear that the architect had a particular love for the geometry of Euclid, as well as a flair for design.     

www.sdflags.com 


















Symmetry

The circular part of each of the round windows shows some measure of symmetry. Thus, one might rotate window II through an angle of 2/3 (120°) or 4/3 (240°), and the window would appear to be unchanged. We say that each of these rotations maps the window onto itself. Reflecting the window in a line along any of the three straight bars again maps the window onto itself. If we count ‘leaving the window as it is’ (or equivalently, rotating the window through an angle of 2 (360°)), we see that there are six ways of mapping the window onto itself.

 Investigate  How can windows IX and VI be mapped onto themselves? How many ways can you find?

 Project  Use your library to learn more about the mathematical concept called a group. The symmetries of Window II form the symmetric group S3. The book The Fascination of Groups by F. J. Budden (Cambridge) is especially good.




















The deltoid

Window XVI contains a non-convex shape made up of three circular arcs called a deltoid.

 Investigate  Beginning with a circle, show how to construct a deltoid. Now draw a tangent to the circle at each vertex of the deltoid, forming an equilateral triangle. If the circle has radius 1 unit, what is the radius of each arc of the deltoid? Can you find the area of the deltoid?

In his book, A Book of Curves (Cambridge), Lockwood gives several different ways for constructing a deltoid.







The coat of arms of the town of Hauterive.





























The Reuleaux triangle

Two of the round windows (IV and XII) contain the shape known as the Reuleaux triangle. Starting with an equilateral triangle, we draw three arcs each with centre at a vertex and radius the side-length of the triangle.

 Investigate  If the triangle has side-length 1, show that the area of the Reuleaux triangle is ( – 3)/2. Show too that the perimeter is .

The Reuleaux triangle is a curve which has constant width: it can be rotated between two fixed parallel tangent lines. There are many such curves; the circle is the simplest and best-known.

Since a Reuleaux triangle has constant width, it can be rotated inside a square so that it always touches the four edges. This idea has been used to construct a drill which will drill square holes!

Two arcs of the Reuleaux triangle give the characteristic Gothic arch; surprisingly, the triangle itself seldom features in Gothic architecture.   

























Straightedge and compass constructions

Straightedge and compass constructions

The early Greeks were very interested in the problem of constructing regular shapes using only straightedge and compass.

 Investigate  Show how an equilateral triangle, a square, and a regular hexagon can be constructed, just using a straightedge and a compass.

Window VIII shows a regular five sided polygon called a pentagram. This pretty shape can also be constructed using just straightedge and compass. The early Greeks would have been pleased!

 Investigate  Try the following construction of the edge P0P1 of a regular pentagon inscribed in a circle having centre O. Take P0 on the circle. Draw radius OBOP0. Join P0 to D, the midpoint of OB. Bisect ODP0 to obtain point N1 on OP. Draw N1P1OP0 to obtain P1 on the circle. Now use your compass to mark off the vertices of the pentagon around the circle.























Fermat primes

Gauss (1777 – 1855) found that a regular polygon having n sides can be constructed using just straightedge and compass if the odd prime factors of n are distinct Fermat primes, that is, prime numbers of the form 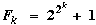. The only known primes of this kind are F0 = 3, F1 = 5, F2 = 17, F3 = 257 and F4 = 65537. The regular 257-gon was constructed in 1832. The mathematician J. Hermes spent ten years constructing the regular 65537-gon, and deposited the manuscript in a large box in the University of Göttingen where it may still be found!

It follows that the first few regular polygons of n sides which can be constructed with straightedge and compass are those with n = 3, 4, 5, 6, 8, 10, 12, and 15. Of course the architect of Hauterive would not have known of Gauss’s result. However, it is more than likely that he was aware of Euclid’s Elements, and in particular Book IV in which Euclid discusses how to inscribe a regular n-gon in a circle for n = 3, 4, 5, 6, and 15.

Strangely, window XVIII gives an implicit 9-gon. However, the window contains the numbers 3 and 5. Perhaps the designer was reaching for the 15-gon?  Or just creating a beautiful design?!  In any case, Hauterive is a fine example of the interplay between mathematics and architecture.

 Link  http://www.jakobsweg.ch/JWE/Weginhalte/WestE/ED2/ejwtd2s1.htm