STELLA OCTANGULA

    The tetrahedron dual

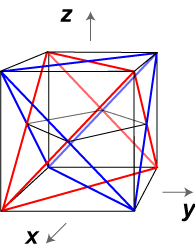
If we construct the dual of a regular tetrahedron by constructing the mediators of the edges, we obtain another congruent tetrahedron. The two tetrahedra fit together in a nice symmetric way as shown.

Show red

Show blue

     

• (a) Make a table of the numbers V, E and F of vertices, edges and faces of the red tetrahedron.

V	E	F

    (b) Now make a table of the numbers V_D, E_D and F_D of vertices, edges and faces of the blue dual solid.

VD	ED	FD

    (c) The situation here is rather trivial, but can you explain why the numbers in (b) occur? Try to establish a correspondence between the numbers in (a) and the numbers in (b).

We notice that in the construction

• the number of red edges is the same as the number of blue edges, since there is a one-to-one correspondence between them;
• the number of red vertices is the same as the number of blue faces, since a red vertex pyramid sits on each blue face;
•  the number red faces is the same as the number of blue vertices, since each red face acts as a base plane for a blue vertex pyramid.

Because the values of V and V_D ( = 4), E and E_D ( = 6), F and F_D ( = 4) coincide, and the vertex and face values are also equal, it is difficult to discern a general pattern here from the numbers. The above argument indicates that here we might logically expect V = F_D, E = E_D, and F = V_D. Since the dual of the regular tetrahedron is again a regular tetrahedron, we say that the tetrahedron is self-dual. We could write: the dual of {3, 3} is again {3, 3}.

The illustrated polyhedron is called the stella octangula. It is the compound of a regular tetrahedron and its dual.

• In the compound polyhedron, does Euler’s formula hold? Look at the diagram above, and count the number of vertices, edges and faces. Notice that these numbers are different from just multiplying the corresponding V, E and F for the tetrahedron by 2. Fill in the following table:

V	E	F	V – E + F

Now check your answers.

  Making the model

Although this is a very simple compound, it makes an attractive model. For nets and instructions on making this model, check out this link.

  Playing with the applet

Spend some time playing with the applet, and enjoying this beautiful compound polyhedron. Use the applet to check out the following statements:

• The stella octangula can be constructed by suitably joining the vertices of a cube.
• The two tetrahedra making up the stella octangula have parallel faces.
• There is a projection of the stella octangula comprising two equilateral triangles (the star of David).
• The stella octangula has three square cross-sections.
•  The intersection of the two tetrahedra is a regular octahedron.

All the above statements are true. If we take all pairs of points in a given set S, and connect them with straight line segments, then the set of points of S together with all the points on the line segments form a convex set C called the convex hull of S. Of course, if S is convex, then it coincides with its convex hull. In the case above, the cube C is the convex hull of the stella octangula S. The three square cross sections are mutually orthogonal, and determine the octahedral intersection. We could think of forming the stella octangula by starting with this octahedron, and adding a triangular pyramid to each face. Another way of thinking of this, is to extend the faces within their face planes until they meet with adjacent extended faces. This is an example of stellation which we will consider later.




  Vertex coordinates

Using the construction with the vertices of the stella octangula occurring as vertices of the encompassing cube, it is easy to see that the contributing tetrahedra can have vertex coordinates.

Now check your answers ...

Use your knowledge of the coordinates of the cube to find the coordinates of
(a) the red tetrahedron, (b) the blue tetrahedron. What now are the coordinates of the edge midpoints?

Since we can take the cube to have vertex coordinates (1, 1, 1), we easily obtain for the tetrahedra,

                                (1, 1, 1),  (–1, –1, 1), (1, –1, –1),  (–1, 1, –1)

and
                                (–1, –1, –1),  (1, 1, –1), (–1, 1, 1),  (1, –1, 1).

The points where the edges of the two tetrahedra meet are the centres of the square faces of the cube:

(1, 0, 0), (0, 1 , 0), (0, 0, 1).

  Historical background

Johannes Kepler, who lived from 1571 to 1630 was a famous German astronomer and mathematician. He is most famous for being the first person to correctly explain planetary motion, thereby becoming the founder of celestial mechanics. Kepler also published work on the regular solids. This included recognition of duality and the idea of a combination of two tetrahedra which he called a stella octangula. This was described in his Harmonices Mundi (1619), the name being Kepler's Latin term for an eight-pointed star. It is both the simplest regular polyhedral compound, and the simplest non-convex uniform polyhedron – that is, a polyhedron with vertices all alike.

  Real life occurrences

The title ‘real life’ is becoming rather a misnomer, but here are some unexpected occurrences.

Example 1
Example 2
Example 3
Example 4

  References