|
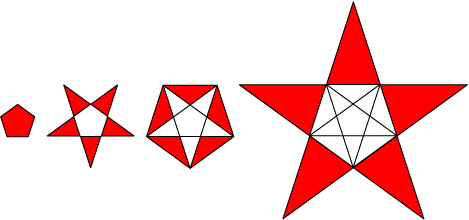
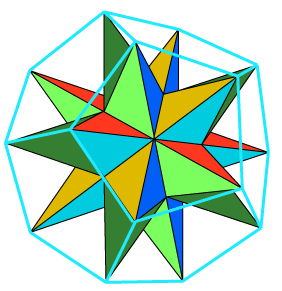
{5/2, 3} or (5/2)3 Our next Kepler-Poinsot polyhedron is the great stellated dodecahedron, illustrated at right. It has intersecting pentagram faces (indicated by the ‘5/2’ in the symbol), and each vertex figure is a triangle {3}. To understand the structure of this solid better, we observe that the polyhedron is inscribed in a regular dodecahedron. As the name indicates, this is also a stellation of the dodecahedron – in fact, the third and final stellation. We shall look at this shortly. Notice that the faces here are intersecting regular pentagrams. These are inscribed in the enclosing dodecahedron. Here are some examples: We are now going to determine the number of vertices, edges and faces. As we have already noted, it is the whole pentagrans that make up the faces here, not the individual parts. Similarly, the vertices of the solid are where the (three) vertices of the pentagram faces meet. And the edges are the long (mostly not completely visible) line segments joining the vertices.
Taking on trust that this a stellation of the dodecahedron, each pentagram face is centred on the face of the inscribed 12-faced regular dodecahedron, so F = 12. And since the vertices coincide with the vertices of a regular dodecahedron, the number of vertices of this solid is 20. For the edges, each of the 12 faces has five edges, giving a count of 5 x 12; since each edge is counted twice in this calculation, we deduce that E = 30. We may be relieved that the Euler number 2 reappears here!
We saw previously how the small stellated dodecahedron and the great dodecahedron can be obtained from a dodecahedron by ‘stellation’ – extending the faces until they meet again, so forming the new solid. The great stellated dodecahedron can also be obtained by stellation: starting with the great dodecahedron, we can extend the faces to obtain this new solid. Another way of saying this is to call the great stellated dodecahedron the third stellation of the dodecahedron. It is in fact the final such stellation.
The figure for the great stellated dodecahedron should in fact be larger than shown in comparison with the preceding figures. Think of each pentagonal face of the great dodecahedron being extended to form a pentagram star. In this way, each dimple of the great dodecahedron is replaced by a triangular pyramid. If we consider the faces of the successive stellations, there is an interesting pattern sequence:
This sequence has an obvious next entry. You might like to think about why it does not appear as the face of a ‘fourth stellation of the dodecahedron’.
The Java applet for this solid is very pretty to play with.
The model of the small stellated dodecahedron is easy to make. See this construction page for some details.
It is a simple matter to derive a set of vertex coordinates for the great dodecahedron. For, as we have seen, these vertices are just the vertices of a regular dodecahedron. Hence from our previous work, we can take the 20 vertex coordinate sets to be: (
MathWorld : http://mathworld.wolfram.com/GreatStellatedDodecahedron.html Wikipedia : http://en.wikipedia.org/wiki/GreatStellatedDodecahedron
Dodecahedron stellations : http://fortran.orpheusweb.co.uk/Poly/Ex/dodstl.htm http://www.ac-noumea.nc/maths/amc/polyhedr/stell_dod_.htm |
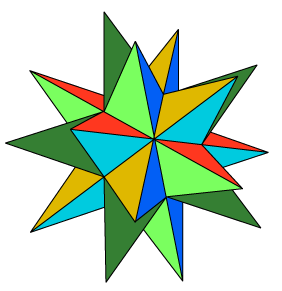 GREAT STELLATED
GREAT STELLATED