 |
A problem of painting |
|
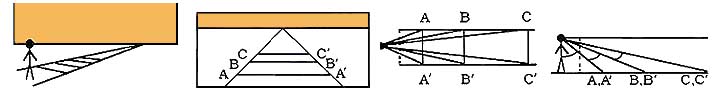
Imagine you are standing on the Nullabor Plain in Australia looking along the railway line. You know that the lines are parallel, yet they appear to meet at a point at eye-level at the horizon. Equally spaced sleepers (ties) appear to rise, and get smaller and closer together the further away they are. Why is it so? The simple geometry of the diagrams below suggests an answer. Now suppose you wish to draw a realistic picture of the scene. This is the problem which has faced artists for thousands of years: how to represent the 3-dimensional world on a 2-dimensional wall or canvas. |
|
 |
|
